International Journal Of Scientific & Engineering Research, Volume 3, Issue 8, August-2012 1
ISSN 2229-5518
Modeling and Simulation of Ethyl Acetate Reactive
Distillation Column Using Aspen Plus
Sohail Rasool Lone & Syed Akhlaq Ahmad
Abstract–– This work presents the modeling and simulation of ethyl acetate reactive distillation column using Aspen Plus TM simulation software. Reactive distillation is a process where simultaneous chemical reaction and vapour -liquid phase separation take place. This represents an exciting alternative to traditional liquid phase chemical reaction processing. In reactive disti llation, separation of products from unconverted reactants allows for greater conversion, because product removal displaces equilibrium and forces the reaction to wards completion. The combination of reaction and distillation helps in achieving products of higher purity and higher conversion of reactants as compared to old conventional processes. Both reaction and distillation acting simultaneously offers certain advantages that c an’t be achieved
by conventional processing. The Aspen PlusTM simulator was used and RADFRAC model was used in Aspen Plus TM which is meant for the
reactive distillation simulation. Reactive distillation is also important from the economic point of view as it involves chem ical reaction and distillation in the same unit and hence it is preffered over the old conventional processes which involve chemical reaction and distillation separately.
—————————— ——————————
REACTIVE distillation involves simultaneous chemical reaction and distillation. The chemical reaction usually takes place in the liquid phase or at the surface of a solid catalyst in contact with the liquid phase. General application of reactive distillation is the separation of close boiling or azeotropic mixtures. A second application of reactive distillation involves taking into account undesirable reaction that may occur during distillation, but the most interesting application involves combining chemical reaction and separation by distillation in a single distillation unit. This technique offers a key opportunity for improving the structure of a process. It is a so-called hybrid process i.e.it merges two different unit operations in a single apparatus namely reaction and distillation. But the combination of distillation and reaction is possible only if the conditions of both unit operations can be combined. Reactive distillation can be used with a wide variety of chemistries including acetylation, aldol condensation, alkylation, amination, dehydration, esterification, etherification, hydrolysis, isomerization, oligomerization, transesterification. Reactive distillation, combination of chemical reaction and distillation in a single unit, has proven to be advantageous over conventional process systems consisting of separate reactor and distillation unit. This concept appears to have been first pronounced by Backhaus, who, starting in 1921,
————————————————
![]() Sohail Rasool Lone, is presently Pursuing M. Tech. in Chemical
Sohail Rasool Lone, is presently Pursuing M. Tech. in Chemical
Engineering from Aligarh Muslim University, Aligarh, email:
lonesohail92@gmail.com.
obtained a series of patents for esterification reaction in a distillation column. This concept of continuous and simultaneous chemical reaction and distillation in a single vessel was also experimentally verified by Leyes and Othmer, for the esterification of acetic acid with an excess of n-butanol in the presence of sulphuric acid as catalyst to produce butyl acetate and water. Further, research both of an experimental and theoretical nature, was conducted during later years. The combination of reaction and distillation over a catalyst bed has been extensively investigated. The most typical examples are MTBE(methyl tert-butyl ether) and cumene production. Modeling of reactive distillation has received considerable attention over the last 15 years and several key contributions have appeared in the literature(Doherty and Malone and the excellent overview of Noeres et al). Pilavachi et al. presented an extensive discussion of several important aspects that affect the accurate modeling of reactive distillation processes. Schenk et al. described in considerable details a hybrid-modeling environment in which a reactive distillation process can be simulated using a combination of equilibrium and mass transfer models, both in steady state and dynamic modes.
Increased speed and improved efficiency.
Lower costs,reduced equipment use, energy use
and handling.
Lesser wastes and fewer by-products.
Improved product quality-reducing opportunity for
degradation, because of less heat, heat duty can be
IJSER © 2012
International Journal Of Scientific & Engineering Research, Volume 3, Issue 8, August-2012 2
ISSN 2229-5518
reduced by utilizing the heat of reaction (if present)
in situ.
Recycle costs for excess reactant, which is necessary
for a conventional reactor to prevent side reactions
Assuming that the streams leaving a stage are in phase equilibrium and that each stream is modelled by an equation of state,we have:
and chemical equilibrium limitation, can be
fi , j
ln( xi , j i , j ) ln( xi , j i , j ) 0
(1.2)
![]()
eq I I II II
reduced.
Reaction conversions can be increased by
Where
xI and
I
![]()
i, j are the mole fraction and fugasity
overcoming chemical equilibrium limitation through the removal of reaction products.
Limitation of azeotropic mixture can be overcome
coefficient of component ‘i’ in stream I of stage ‘j’
respectively.The energy balance equation is:![]()
h II I I II
by reaction, described by Terril et al.
fi, j
(Rj
1)H j
I
(Z j
1)H j
II
(H j 1
H j 1
HFj
Qj ) 0
(1.3)
Where H j
and H j
are the total enthalpy flows of streams
I and II to the subsequent stages , Q j is the heat load in each
Each stage is a perfectly mixed i.e. liquid
stage and
H Fj is the enthalpy flow of the feed stream to
composition at each stage is homogeneous and equal to the composition of liquid leaving the stage.
The vapour and liquid leaving any stage are in physical equilibrium.
Entrainment of liquid drops in vapour and occlusion of vapour bubbles in liquid are negligible.
The heats of reaction are negligible.
stage ‘j’.All enthalpy values are reffered to the pure elements
at 298.15 K,1 bar.
At the top and at the bottom, we have the condenser and the reboiler respectively.We need to add one more equation associated to reflux flow in the column:![]()
nc nc
lv I II
(1.4)
The reactions take place in the bulk liquid.
Vapour molar holdup and vapour-phase chemical
f j (Z j
1) ni , j
i 1
E j ( R j
1) ni , j 0
i 1
reactions are neglected.
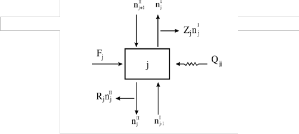
The objective of this work was the modeling and simulation of ethyl acetate reactive distillation column in steady state operation. The configuration of each stage is
Variable E j is specified for the condenser and the reboiler , and is calculated for the inner stages. Equation (1.4 ) is used for the total or partial condensers and reboilers, depending on the values specified for R j , Z j and E j .
The chemical equilibrium equation for each reaction ‘k’ in stage ‘j’ is:![]()
nc
shown in figure 1.The mass balance of component ‘i’ in a
stage ‘j’ is given by:![]()
nr
vi ,k i , j 0
i 1
(1.5)
m II I II I
fi, j
(Rj
1)ni, j
![]()
(Z j
I
1)ni , j
(ni , j 1
ni , j 1
Fi , j
vi ,k
k 1
) 0 (1.1)
k , j
At chemical and phase equilibrium, the chemical potential of a component is the same in the liquid and
Where (Z j
1)ni. j
is the molar flow rate of component ‘i’
I
vapour phases. We arbitrarily chose to write the chemical
that leaves stage ‘j’ as vapour , Z j ni , j
the flow rate in the
equilibrium equation using the chemical potentials of phase![]()
vapour side stream and ni, j
is the flow rate to the I:
subsequent stage. (Rj
1)ni, j
is the molar flow rate of
II
![]()
component ‘i’ that leaves the stage ‘j’ as liquid , Rj ni , j
the
0,ig I I
flow rate in the liquid side stream and nII is the flow rate to
i , j i , j
(Tj , P0 )
RTj ln(xi , j i , j Pj / P0 )
(1.6)
the subsequent stage. Fi , j is the molar flow rate of the feed
We use
0,ig
![]()
P0 =1 bar and Pj
in bar. In equation (1.6),![]()
stream to stage j. In this equation, vi ,k is the stoichiometric
i, j
(Tj , P0 ) is the molar Gibbs free energy of formation of
coefficient of component ‘i’ in the reaction ‘k’,
k , j the extent
pure component ‘i’ at temperature T j
and pressure P0 in
of reaction ‘k’ in tage ‘j’ and ‘nr’ is the number of
the idea gas state. Combining equations (1.5) and (1.6),and
independent chemical reactions.
the definitions of mole fractions ,and dividing by
obtain the chemical equilibrium equation as under:
RT j ,we
![]()
nc nc nc
f r [
v ( 0,ig (T , P ) / RT )
v ln(nI I P / P nI )] 0
k , j i ,k i , j j 0
i 1
j i ,k i , j i , j j
i 1
0 i , j
i 1
(`1.7)
This form of the chemical equilibrium equation (Equation(1.7)) is particularly convenient for it’s easy differentiation.
Fig.1 Configuration of each stage
IJSER © 2012
International Journal Of Scientific & Engineering Research, Volume 3, Issue 8, August-2012 3
ISSN 2229-5518

The esterification of acetic acid with ethanol towards ethyl acetate and water occurs according to the reaction.
CH3COOH C2 H5OH CH3COOC2 H5
H 2O
The model of the reaction rate use in this work is as under:
r k1C1C2
k2C3C4
(1.8)
The rate constants
k1 and
k 2 are given as under:
k1 4.76 10 exp( 59774( J / gmol ) / RT )
(1.9)
k 1.63 10 4 exp( 59774( J / gmol) / RT )
(1.10)
![]()
![]()

Aspen PlusTM was used for simulating the above model.In the Aspen PlusTM ,there is an inbuilt model known as RADFRAC which is meant for the simulation of the reactive distillation columns.In the example that has been mentioned above i.e. the esterification of acetic acid with ethanol towards ethyl acetate and water, the column has eight stages(reboiler, six adiabatic plates and condenser).The numbering of stages is done bottom upward and column pressure is taken as one atmosphere. All the stages are reactive stages. The feed rate is 4.3067 kmol/sec with a liquid distillate of 0.7083 kmol/sec. The feed is saturated liquid at feed tray pressure and the reflux ratio is 2.1 and boilup ratio is 0.5945.The condenser is a total condenser and reboiler is a partial reboiler. The feed is feed to the third stage from the top and has the mole fraction compostion as: acetic acid
Fig 2 Temperature Profile in Ethyl Acetate Production
x1 0.2559 ,ethanol
x3 0.0539 and water
x2 0.6159 ,ethyl acetate
x4 0.0743 .Holdup volumes are
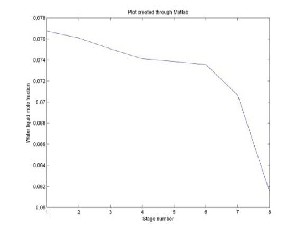
Fig. 3 Acetic Acid liquid mole fraction in Ethyl Acetate
0.6 and 0.4 litres, respectively for reboiler and each of the
stages 1-7.The Aspen PlusTM simulator was used in this
work and using the above given specifications in the
RADFRAC model which is meant for the simulation of
reactive distillation columns. There are a number of
thermodynamic property models in Aspen PlusTM which can
be used for the estimation of various thermodynamic
properties, but Wilson property model was in this work. The
Wilson model is very much accurate for the present system,
and hence it was used. The results that were obtained upon
the simulation of the model are given in the form of graphs
below.
IJSER © 2012
Production
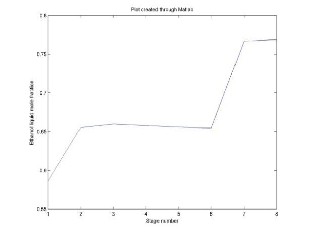
Fig.4 Ethanol liquid mole fraction in Ethyl Acetate
Production
International Journal Of Scientific & Engineering Research, Volume 3, Issue 8, August-2012 4
ISSN 2229-5518

of reactants as compared to old conventional processes. The mathematical model developed has shown satisfactory results in simulating a reactive distillation column for the esterification of acetic acid with ethanol to produce ethyl acetate. This type of equipment was selected for the study, because it represents a complex unit operation, due to the simultaneity of reaction and separation.
Fig.5 Ethyl Acetate liquid mole fraction in Ethyl Acetate
Production
This work was supported by Aligarh Muslim University,
Aligarh(UP)-India,202002.I am very much thankful to Dr. Syed Akhlaq Ahmad, Associate Professor, Department of Chemical Engineering, AMU, Aligarh, for guiding me in Aspen Plus Simulation of this work.
m
i, j
Mass balance function of component i in stage j.
f eq Phase equilibrium function of component i in stage j.
R j Liquid side stream fraction at stage j.
Z j Vapor side stream fraction at stage j.
Fig.6 Water liquid mole fraction in Ethyl Acetate
Production
The above results show a qualitative agreement with the experimental data. However, the small difference between the values of the calculation and those of the experimental data may be due to some aspects of thermodynamic modeling. In this system, ideal vapour phase is a strong simplifying assumption, because acetic acid molecules are known to dimerize in the vapour phase causing considerable deviations from the ideal gas behaviour.
In this work, all the results were obtained for steady-state using Aspen PlusTM for ethyl acetate reactive distillation column. The combination of reaction and distillation helps
in achieving products of high purity and higher conversion
nI Molar flow rate of component i in stream I of stage j.
Fi , j Molar flow rate of the stream of component i to stage j.
T j Temperature at stage j.
E j Relation between the liquid and vapour streams.
xI Mole fraction of component i in stream I of stage j.
R Universal gas constant.
vi ,k Stoichiometric coefficient of component i in reaction k.
![]()
i , j Chemical potential of compound i at stage j.
I
![]()
i , j Fugacity coefficient of component i in stream I of stage
j.
II
![]()
i, j Activity coefficient of component i in stream II of stage
j.![]()
i ,k Kinetic order of component i in reaction k.
IJSER © 2012
International Journal Of Scientific & Engineering Research, Volume 3, Issue 8, August-2012 5
ISSN 2229-5518
[1] Aspen Plus help manual, Aspen Tech., 2006.
[2] A.K.Jana, ‚Process Simulation and Control using Aspen Plus‛.
[3 C.D. Holland, ‛Fundamentals of Multicom- ponent Distillation‛,
New York, McGraw Hill,1981.
[4] J.D. Seader & Ernest J. Henley, ‚Separation Process Principles‛, 2nd
Edition, Wiley India Pvt.Limited, 2010.
[5] Marcelo F. Alfradique & Marcelo Castier, ‚ Modeling and Simulation of Reactive Distillation Columns using Computer Algebra‛, Computers and Chemical Engineering,29, 1875-
1884,2005.
[6] J.D. Seader & Ernest J. Henley, ‚Separation Process Principles‛, 2nd
Edition, Wiley India Pvt.Limited,2010.
[7] Stichmair J.G & Frey T., ‚ Reactive Distillation Processes‛,
Chemical and Engineering Technology,22,95-103,1999.
IJSER © 2012